The candidate will be selected on the basis of Admission Test and the Test shall comprise questions of English, Science (Physics, Chemistry, Biology), General Knowledge and Mathematics.
BIOLOGY (BOTANY)
An Introduction to Botany
The living world nature and scope of biology, characteristics of living organisms, differences between plants and animals and taxonomic categories.
Biological classification two kingdom classification and its short comings, five kingdom classification, kingdom Monera, kingdom Protista, kingdom Fungi, kingdom Plantae, kingdom Animalia and viruses, viroids, lichens and mycorrhizae.
Plant kingdom algae, bryophytes, pteridophytes, gymnosperms, angiosperms and plant life cycles and alternation of generations.
Structural Organization in Plants
Morphology of flowering plants the root, the stem, the leaf, the inflorescence, the flower, the seed, semi-technical description of some important familities (Fabaceae, Solanaceae, Liliaceae).
Anatomy of flowering plants the tissues, the tissue system, anatomy of dicotyledonous and monocotyledonous plants and secondary growth.
Structure and Functions
The plant cell cell theory, structural organization (organelles and their function), prokaryotic and eukaryotic cells, cell wall, biomembranes and fluid mosaic model.
Biomolecules primary and secondary metabolites, proteins, polysaccharides, nucleic acids, concept of metabolism and enzymes.
Cell cycle and cell division cell cycle, mitosis and meiosis and their significance.
Plant Physiology
Transport in plants means of transport, plant water relations, long distance transport of water, transpiration, uptake and transport of mineral nutrients and phloem transport flow from source to sink.
Mineral nutrition modes of nutrition, essential mineral elements and their major functions, mechanism of absorption of elements and metabolism of nitrogen.
Photosynthesis in higher plants early experiments, seat of photosynthesis, pigments involved in photosynthesis, light reaction dark reaction, the C4 pathway and photorespiration.
Respiration in plants gas exchange in plants, glycolysis, fermentation, aerobic respiration, Krebs cycle, electron transport system and oxidative phosphorylation.
Plant growth and development growth, differentiation, development, major plant growth regulators, photoperiodism and vernalization.
Reproduction
Reproduction in organisms asexual reproduction and sexual reproduction.
Sexual reproduction in flowering plants structure of flower, pollination, fertilization, apomixes and ployembryony.
Genetics and Evolution
Principles of inheritance and variation Mendels laws of inheritance, inheritance of one gene, inheritance of two genes, sex determination, mutation and genetic disorders.
Molecular basis of inheritance nucleic acids, replication, transcription, genetic code, translation, regulation of gene expression, DNA fingerprinting, gene pools and genetic conservation.
Evolution origin of life, evolution of life forms : a theory, evidences for evolution and mechanism of evolution.
Biology (Botany) In Human Welfare
Strategies for enhancement in food production plant breeding and plant introduction, use of fertilizers (economic and ecological aspects), use of pesticides (advantages and hazards), single cell proteins, and tissue culture and its application.
Microbes in human welfare microbes in household products, industrial products, sewage treatment, production of biogas and microbes as biocontrol agents and as biofertilisers, green manure, crop residues and nitrogen fixation (symbiotic and nonsymbiotic).
Biotechnology
Biotechnology : principles and processes principles of biotechnology, tools of recombinant DNA technology and processes of recombinant DNA technology.
Biotechnology and its application biotechnological applications in agriculture and medicine, and ethical issues.
Ecology
Organisms and population organisms and its environment and populations.
Ecosystem ecosystem structure and function, energy flow, food chain and food web, ecological pyramids, ecological succession and nutrient cycling.
Biodiversity and conservation biodiversity and its conservation.
Environmental issues air, noise and water populations and their control, solid wastes, agro-chemicals and theirs effects, radioactive wastes, green house effect and global warning, eutrophication, ozone depletion, degradation by improper resource utilization, conservation of natural resources and deforestation.
Zoology
- Diversity of living organisms, Classification of the living organisms (Five Kingdom Classification major groups and principles of Classification within kingdom : Protista and Animalia). Systematics and binomial system of nomenclature, Salient features of animal classification (nonchordates up to Phylum level and chordates up to Class level), Zoological Parks and Museum.
- Tissues in animals, Morphology, anatomy and functions of different systems of an annielid (earthworm), an insect (cockroach) and an amphibian (frog).
- Basic chemical constituents of living bodies. Structure and function of carbohydrates, proteins, lipids and nucleic acids, Enzymes : Types, properties and functions.
- Digestion and absorption, Breathing and Respiration, Body fluids and circulation, Excretory-products and elimination, Locomotion and movement, Control and Coordination, Reproductive system in male and female, menstrual cycle, production of gametes, fertilization, implantation, embryo development, pregnancy parturition and lactation, Reproductive Health : birth control, contraception and sexually transmitted diseases, infertility.
- Human Genetics : Sex determination in human being : XX, XY, Linkage and Crossing over. Inheritance pattern of haemophilia and blood groups Aligarh Muslim University in human beings, Genome and Human Genome Project, DNA fingerprinting.
- Theories of organic evolution, Evidences and Mechanism of organic evolution.
- Animal Husbandry, Basic concept of immunology, vaccines, Pathogens, parasites, Cancer and AIDS, Adolescence and drug / alcohol abuse, Recombinant DNA technology.
- Species population and community, Animal ecological adaptation, Centres of diversity and conservation of biodiversity, National parks and Sanctuaries.
Chemistry
Syllabus Chemistry for All Competitive Admission Tests With Class XII As Eligibility
- Some Basic concepts of Chemistry, Structure of Atom, Classification of elements and periodicity in properties, Chemical bonding and molecular structure.
- States of matter : Gases and liquids, Solid State, Solutions.
- Thermodynamics.
- Equilibrium, Redox reactions, Electrochemistry.
- Chemical Kinetics, Surface Chemistry.
- Hydrogen, General principles and process of isolation of elements, Studies of s & p-d and f block elements, Coordination compounds.
- Organic Chemistry : Some basic principles and Techniques, Hydrocarbons. Haloalkanes and Haloarenes, alcohols, phenols and Ethers.
- Aldehydes, Ketones and Carboxylic acids.
- Organic compounds containing nitrogen.
- Biomolecules, Polymers, Chemistry in everyday life.
- Environmental Chemistry.
Note : Prescribed Book : Text Books of Chemistry Class XI and Class XII NCERT Publication, latest edition.
Physics Syllabus of Admission Tests for Admission to BUMS / MBBS & BDS / B. TECH. & B. ARCH. / CET / BCA / GEN. NURSING
PHYSICS SYLLABUS of Diploma in General Nursing
Undergraduate Physics Syllabus for Admission Test Mechanics
Physical World and Measurement
Physics scope and excitement, nature of physical laws; Physics, Technology and society. Need for measurement : Units of measurement; systems of units; SI units, fundamental and derived units, Length, mass and time measurements; accuracy and precision of measuring instruments, errors in measurement; significant figures.
Dimensions of physical quantities, dimensional analysis and its applications.
Kinematics
Frame of reference, Motion in a straight line : Position-time graph, speed and velocity, Uniform and non-uniform motion, average speed and instantaneous veolocity. Uniformly accelerated motion, velocity-time, position-time graphs, relations for uniformly accelerated motion (graphical treatment).
Elementary concepts of differentiation and integration for describing motion. Scalar and vector quantities : Position and displacement vectors, general vectors and notation, equality of vectors, multiplication of vectors by a real number; addition and subtraction of vectors. Relative velocity.
Unit vector; Resolution of a vector in a plane-rectangular components. Motion in a plane. Cases of uniform velocity and uniform acceleration projectile motion. Uniform circulation motion.
Laws of Motion
Intuitive concepts of force. Inertia, Newtons first law of motion; momentum and Newtons second law of motion; impulse; Newtons third law of motion. Law of conservation of linear momentum and its applications.
Equilibrium of concurrent forces, static and kinetic friction, laws of friction, rolling friction. Dynamics of uniform circular motion: Centripetal force, examples of circular motion (vehicle on level circular road, vehicle on banked road).
Work, Energy and Power
Scalar product of vectors. Work done by a constant force and a variable force; kinetic energy, work-energy theorem, power.
Notion of potential energy, potential energy of a spring, conservative forces : conservation of mechanical energy (kinetic and potential energies); nonconservative forces : elastic and inelastic collisions in one and two dimensions.
Motion of System of Particles and Rigid Body
Centre of mass of a two-particle system, momentum conversation and centre of mass motion.
Centre of mass of a rigid body; centre of mass of uniform rod.
Vector product of vectors; moment of a force, torque, angular momentum, conservation of angular momentum with some examples.
Equilibrium of rigid bodies, rigid body rotation and equiations of rotational motion. Comparison of linear and rotational motions; moment of inertia, radius of gyration.
Values of moments of inertia for simple geometrical objects (no derivation). Statement of parallel and perpendicular axes theorems and their applications.
Gravitation
Keplars laws of planetary motion. The Universal law of gravitation.
Acceleration dues to gravity and its variation with altitude and depth.
Gravitational potential energy; gravitational potential, Escape Velocity, Orbital Velocity of a satellite, Geo-stationary satellites.
Properties of Bulk Matter
Elastic behaviour, Stress-strain relationship, Hookes law, Youngs modulus, bulk modulus, shear, modulus of rigidity.
Pressure due to a fluid column; Pascals law and its applications (hydraulic lift and hydraulic brakes). Effect of gravity on fluid pressure.
Viscosity, Stokes law, terminal velocity, Reynolds number, streamline and turbulent flow, Bernoullis theorem and its applications.
Surface energy and surface tension, angle of contact, application of surface tension ideas to drops, bubbles and capillary rise.
Heat, temperature, thermal expansion; specific heat-calorimetry; change of statelatent heat. Heat transfer-conduction, convection and radition, thermal conductivity, Newtons law of cooling.
Thermodynamics
Thermal equilibrium and definition of temperature (zeroth law of thermodynamics). Heat, work and internal energy. First law of thermodynamics.
Second law of thermodynamics : reversible and irreversible processes. Heat engines and refrigerators.
Behaviour of Perfect Gas and Kinetic Theory
Equation of state of perfect gas, work done on compressing a gas.
Kinetic theory of gases-assumptions, concept of pressure. Kinetic energy and temperature; rms speed of gas molecules; degrees of freedom, law of Aligarh Muslim University equipartition of energy (statement only) and application to specific heats of gases; concept of mean free path, Avogadros number.
Oscillations and Waves
Periodic motion-period, frequency, displacement as a function of time. Periodic functions. Simple harmonic motion (S.H.M.) and its equation; oscillations of a spring restoring force and force constant; energy in S.H.M. kinetic and potential energies simple pendulum derivation of expression for its time period free, forced and damped oscillations(qualitative ideas only), resonance.
Wave motion, Longitudinal and transverse waves, speed of wave motion. Displacement relation for a progressive wave. Principle of superposition of waves, reflection of waves, standing waves in strings and organ pipes, fundamental mode and harmonics, Beats, Doppler effect.
Electrostatics
Electric charges, Conservation of charge, Coulombs low-force between two point charges forces between multiple charges, superposition principle and continuous charge distribution.
Electric field, electric field due to a point charge, electric field lines electric dipole electric field due to a dipole torque on a dipole in uniform electric field.
Electric flux, statement of gausss theorem and its applications to find field due to infinitely long straight wire uniformly charges infinite plane sheet and uniformly charged tin spherical shell (field inside and outside).
Electric potential difference, electric potential due to a point charge, a dipole and system of charge; equipotential surfaces, electrical potential energy of a system of two point charges and of electric dipole in an electrostatic field.
Conductors and insulators free charges and bound charges inside a conductor. Dielectrics and electric polarization, capacitors and capacitance, combination of capacitors in series and in parallel, capacitance of a parallel plate capacitor with and without dielectric medium between the plates, energy stored in a capacitor. Van de Graaff generator.
Current Electricity
Electric current flow of electric chargers in a metallic conductor drift velocity, mobility and their relation with electric current; Ohms electrical resistance, V-I characterstics (linear and non-linear), electrical energy and power, electrical resistivity and conductivity. Carbon resistors colour code for carbon resistors; series and parallel combinations of resistors; temperature dependence of resistance.
Internal resistance of a cell, potential difference and emf of a cell combination of cells in series and in parallel.
Kirchhoffs laws and simple applications. Wheatstone bridge and metre bridge.
Potentiometer principle and its applications to measure potential difference and for comparing emf of two cells; measurement of internal resistance of a cell.
Magnetic Effects of Current and Magnetism
Concept of magnetic field, Oersteds experiment.
Biot-Savart law and its application to current carrying circular loop.
Amperes law and its applications to infinitely long straight wire, straight and toroidal solenoids.
Force on a moving charge in uniform magnetic and electric fields. Cyclotron.
Force on a current carrying conductor in a uniform magnetic field. Force between two parallel current carrying conductors definition of ampere. Torque experienced by a current loop in uniform magnetic field; moving coil galvanometer its current sensitivity and conversion to ammeter and voltmeter. Current loop as a magnetic dipole and its magnetic dipole moment. Magnetic dipole, moment of a revolving electron, magnetic field intensity due to a magnetic dipole (bar magnet) along its axis and perpendicular to its axis. Torque on magnetic dipole (bar magnet) in a uniform magnetic field; bar magnet as an equivalent solenoid magnetic field line; Earths magnetic field and magnetic elements pars dia and ferro magnetic substances, with examples. Electromagnets and factors of affection their strengths. Permanent magnets.
Electromagnetic Induction and Alternating Currents
Electromagnetic Induction; Faradays law, Induced emf and current; Lenzs law, Eddy current self and mutual inductance.
Need for displacement current.
Alternating currents, peak and rms value of alternating; current / voltage, reactance and impedance;
LC oscillations (qualitative treatment only), LCR series circuit, resonance, power in ac circuits wattles current.
AC generator and transformer.
Electromagnetic Waves
Aligarh Muslim University Displacement current, current Electromagnetic wave and their characteristics (qualitative ideas only) Transverse nature of electromagnetic waves.
Electromagnetic spectrum (radio waves, microwaves infrared, visible ultraviolet, x-rays gamma rays) including elementary facts about their uses.
Optics.
Reflection of light spherical mirror, mirror formula refraction of light, total internal reflection and its applications, optical fibres refraction at spherical surfaces, lenses thin lens formula lens makers Formula. Magnification power of a lens, combination of thin lenses in contract. Refraction and dispersion of light through a prism.
Scattering of light blue colour of the sky and reddish appearance of the sun at sunrise and sunset.
Optical instruments : Human eye, image formation and accommodation, correct of eye defects (myopia, hypermetropia, presbyopia and astigmatism) using lenses. Microscopes and astronomical Telescopes (reflecting and refraction) and their magnifying powers.
Waves optics : Wave front and Huygens principle reflection and refraction of plane wave at a plane surface using wave fronts. Proof of laws of reflection and refraction using Huygens principle, Interference, Youngs double slit experiment and expression for fringe width coherent sources and sustained interference of light. Diffraction due to a single slit, width of central maximum. Resolving power of microscopes and astronomical telescopes Polarization, plane polarized light; Brewsters law. Uses of plane polarized light and polaroids.
Dual Nature of Matter and Radiation
Dual nature of Radiation Photoelectric, Hertz and Lenards observations; Einsteins Photoelectric equation particle nature of light.
Master waves wave nature of particles, de Broglie relation. DAvission General experiment.
Atoms & Nuclei
Alpha particle scattering experiment, Rutherfords model of atom; Bohr model, energy levels hydrogen spectrum.
Composition and size of nucleus, atomic masses, isotopes, isobars; isotones. Radioactivity alpha, beta and gamma particles / rays and their properties; radioactive decay law Mass-energy relation, mass defect; binding energy per Aligarh Muslim University nucleon and its variation with mass number, nuclear fission, nuclear reactor, nuclear fusion.
Electronic Devices
Semicondoctors; semiconductor diode I V, characteristics in forward and reverse bias, diode as a rectifier; I V characteristics of LED, photodiode, solar cell and Zener diode : Zener diode as a voltage regulator. Junction transistor, transistor action characteristics of a transistor; transistor as an amplifier (common emitter configuration) and oscillator. Logic gages (OR, AND, NOT NAND and NOR ). Transistor as a switch.
Communication Systems
Elements of a communication system (block diagram only); bandwidth of signals (speech, TV and digital data); bandwidth of transmission medium. Propagation of electromagnetic waves in the atmosphere, sky and space wave propagation. Need of modulation. Production and detection of an amplitude-modulate wave.
Syllabus for Diploma in General Nursing
Mathematics
UNIT I : SETS AND FUNCTIONS
1. SETS (3+3)
Sets and their representations. Empty set. Finite & Infinite sets. Equal sets. Subsets. Subsets of the set of real numbers especially Intervals (with notations). Power set. Universal set. Venn diagrams. Union and Intersection of sets. Difference of sets. Complement of a set.
2. RELATIONS & FUNCTIONS (4+4)
Orders pairs, Cartesian product of sets. Number of elements in the Cartesian product of two finite sets. Cartesian product of the reals with itself (upto R x R x R). Definition of relation, pictorial diagrams, domain, codomain and range of a relation. Function as a special kind of relation from one set to another. Pictorial representation of a function, domain, co-domain and range of a function. Real valued function of the real variable, domain and range of these functions, constant, identity, polynomial, rational modulus, signum and greatest integer functions with their graphs, Sum, difference, product and quotients of functions.
3. TRIGNOMETRIC FUCNTIONS :
Positive and negative angles. Measuring angles in radians and in degrees and conversion from one measure to another. Definition of trigonometric functions with the help of unit circle. Truth of the identity sin2 x + cos2 x = 1 for all x. Signs of trigonometric functions and sketch of their graphs. Expressing sin (x + y) and cos (x+y) in terms of sin x, sin y, cos x and cos y. Deducing the identities like the following :
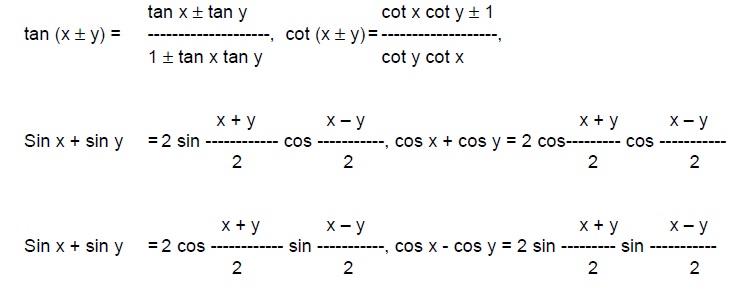
Identities related to sin 2 x, cos 2 x, tan 2 x, sin 3 x, cos 3 x and tan 3 x.
General solution of trigonometric equiations of the type sin q = sin a
UNIT II : ALGEBRA
1. PRINCIPLE OF MATHEMATICAL INDUCTION (03)
Process of the proof by induction, motivating the application of the method by looking at natural numbers as the least inductive subset of real numbers. The principle of mathematical induction and simple applications.
2. COMPLEX NUMBERS AND QUADRATIC EQUATIONS (3+3)
Need for complex numbers, especially -1 , to be motivated by inability to solve every quadratic equation. Brief description of algebraic properties of complex numbers. Argand plane and polar representation of complex numbers. Statement of Fundamental Theorem of Algebra, Solution of quadratic equations in the complex number system.
3. LINEAR INEQUALITIES : (03)
Linear inequalities, Algebraic solutions of linear inequalities in one variable and their representation on the number line. Graphical solution of linear inequalities in two variables. Solution of system mof linear inequalities in two variables graphically.
4. PERMUTATIONS AND COMBINATIONS (04)
Fundamental principle of counting. Factorial n (n1) Permutations and combinations, derivation of formulae and their connections, simple applications.
5. BINOMIAL THEOREM : (08)
History, statement and proof of the binomial theorem for positive integral indices. Pascals triangle, General and middle term in binomial expansion, simple applications.
6. SEQUENCE AND SERIES (06)
Sequence and Series, Arithmetic progression (A > P), arithmetic mean (A.M.) Geometric progression (G.P., General term of a G.P., sum of n terms of a G.P., geometric mean (G > M), relation between A.M. and G.M. Sum to a terms of the special series ?n , ?n2 and ?n3 .
UNIT III : COORDINATE GEOMETRY :
1. STRAIGHT LINES (04)
Brief recall of 2 D from earlier classes. Slope of a line and angel between two lines. Various forms of eqwuations of a line : parallel to axes, point-slope form, slope intercept form, two point form, intercepts form and normal form. General equation of a line. Distance of a point from a line.
2. CONIC SECTION (04)
Sections of a cone : circle, ellipse, parabola, hyperbola, a point, a straight line and pair of intersecting lines as a degenerated case of conoic section. Standard equations and simple properties of parabola, ellipse and hyperbola. Standard equation of a circle.
3. INTRODUCTION TO THREE DIMENSIONAL GEOMETRY (03)
Coordinate axes and coordinate planes in three dimensions. Coordinatoes of a point. Distance between two points and section formula.
UNIT IV : CALCULUS
1. LIMITS AND DERIVATIVES : (04)
Derivative introduced as rate of change both as that of distance function and geometrically, intuitive idea of limit. Definition of derivative, relate it to slope of tangent of the curve, derivative of sum, difference, product and quotient of functions. Derivatives of polynomial and trigonometric functions.
UNIT V : MATHEMATICAL REASONING 1. MATHEMATICAL REASONING (03)
Mathematically acceptable statements. Connecting words / phrases consolidating the understanding of if and only if (necessary and sufficient) condition, implies, and/ or, implied by, and, or, three exists and their use through variety of examples related to real life and mathematics. Validating the statements involving the connecting words difference between contradiction, converse and contrapositive.
2. PROBABILITY : (03)
Random experiments : Outcomes, sample, spaces (set representation). Events : occurrence of events, `not, `and and `or events, exhaustive events, mutually exclusive events Axiomatic (set theoretic) probability, connections with the theories of earlier classes. Probability of an event, Probability of `not, `and and `or events.
RECOMMENDED TEXT BOOKS :
(1) Mathematics Part I Textbook for Class XI, NCERT Publication.
(2) Mathematics Part II Textbook for Class XI, NCERT Publication.
UNIT I RELATIONS AND FUNCTIONS
1. Relations and Functions :
Types of relations : reflexive, symmetric, transitive and equivalence relations. One to one and onto functions, composite functions, inverse of a function. Binary operations.
2. Inverse Trigonometric Functions :
Defintion, range, domain, principal value branches, Graphs of inverse trigonometric functions. Elementary properties of inverse trigonometric functions.
UNIT II : ALGEBRA
1. Matrices :
Concept, notation, order, equality, types of matrices, zero matrix, transpose of a matrix, symmetric and kew symmetric matrices. Addition, multiplication and scalar multiplication of matrices, simple properties of edition, multiplication and scalar multiplication. Non commutativity of multiplication of matrices and existence of non zero matrices whose product is the zero amt4rix (restrict to square matrices of order 2). Concept of elementary row and column operations. Invertible matrices and proof of the uniqueness of inverse, if it exists. (Here all matrices will have real entries).
2. Determinants :
Determinant of a square matrix (upto 3 x 3 matrices), properties of determinants, minors, cofactors and applications of determinants in finding the area of a triangle. A joint and inverse of a square matrix. Consistency, inconsistency and number of solutions of system of linear equations by examples, solving system of linear equations in two or three variables (having unique solution) using inverse of a matrix.
UNIT III : CALCULUS
1. Continuity and Differentiability : Continuity and Differentiability, derivative of composite functions, chain rule, derivative of inverse trigonometric functions, derivative of implicit function. Concept of exponential and logarithmic functions and their derivative. Logarithmic differentiation. Derivative of functions expressed in parametric forms. Second order derivatives. Rolles and Lagranges Mean Value Theorems (without proof) and their geometric interoperations.
2. Applications of Derivatives :
Applications of derivatives : rate of change, increasing / decreasing functions, tangets and normals, approximation, maxima and minima (first derivative test motivated geometrically and second derivative test given as a provable tool).. Simple problems (that illustrate basic principles and understanding of the subject as well as real life situations).
3. Integrals
Integration as inverse process of differentiation. Integration of a variety of functions by substitution, by partial fractions and by parts, only simple integrals of the type :
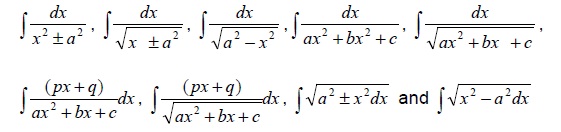
To be evaluated.
Define integrals as a limit of sum, Fundamental Theorem of calculus (without proof). Basic properties of definite integrals and evaluation of definite integrals.
4. Applications of the Integrals :
Applications in findings the area under simple curves, especially lines, areas of circles / parabolas / ellipse (in standard form only), area between the two above said curves (the region should be clearly identifiable).
5. Differential Equations :
Definition, order and degree, general and particular solutions of a differential equation. Formation of differential equation whose general solution is given. Solution of differential equations by method of separation of variables, homogeneous differential equations of first order and first degree. Solutions of linear differential equations of the type :.
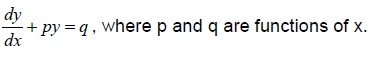
UNIT IV : VECTORS AND THREE DIMENSIONAL GEOMETRY :
1. Vectors : Vectors and scales, magnitude and direction of a vector. Direction cosines / ratios of vectors. Types of vectors (equal, unit, zero, parallel and collinear vectors), position vector of a point, negative of a vector, components of a vector, addition of vectors, multiplication of a vector by a scalar, position vector of a point dividing a line segment in a given ratio Scalar (dot) product of vectors, projection of a vector on a line. Vector (cross) product of vectors.
2. Three dimensional Geometry :
Direction cosines / ratios of a line joining two points. Cartesian and vector equation of a line, coplanar and skew lines, shortest distance between two lines. Cartesian and vector equation of a plane. Angle between (1) two lines, (ii) two planes, (iii) a line and plane. Distance of a point from a plane.
UNIT V : LINEAR PROGRAMMING
1. Linear programming
Introduction, definition of related terminology such as constraints, objective function, optimization, different types of linear programming (LP) problems, mathematical formulation of LP, problems, graphical method of solution for problems in two variables, feasible and infeasible regions, feasible and infeasible solutions, optional feasible solutions (upto three non trivial constraints).
UNIT VI : PROBABILITY
1. Probability : Multiplication theorem on probability, Conditional probability, independent events, total probability, Bayes theorem, Random Variable and its probability distribution, mean and variance of haphazard variable. Repeated independent (Bernoulli) trials and Binomial distribution.
Recommended text books :
1.Mathematics Part I : Textbook for Class XII, NCERT, Publication.
2.Mathematics Part II Textbook for Class XII, NCERT, Publication.
Click Here for All Courses Syllabus Click Here for AMU Home Page


